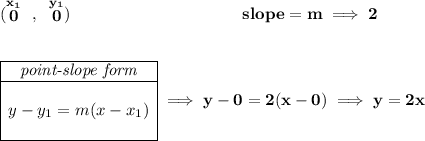
bearing in mind that perpendicular lines have negative reciprocal slopes.
now, they both intersect at 0,0, namely they both pass through it, we know the slope of the first one, so
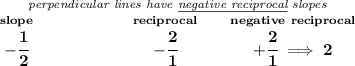
so, we're really looking for the equation of a line whose slope is 2, and runs through (0,0).