Question

The minimum point on the graph of the equation y=f(x) is (−1,−3). What is the minimum point on the graph of the equation y=f(x−5)

Answer 1

Answer: (4,-3)

Explanation:

When we shift a function g(x) , c units to the right , then the new function is given by :-

`g(x-c)`

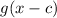
When we compare functions f(x) and f(x-5), we find that f(x-5) is the function which comes after a 5 units rightwards shift in f(x).

Also, The minimum point on the graph of the equation y=f(x) is (−1,−3).

The translation rule to move a point rightwards by d units:-

`(x,y)\rightarrow (x+d,y)`

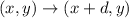
Using the above translation rule , we have

The minimum point on the graph of the equation
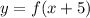`y=f(x+5)` as:

`(-1,-3)\rightarrow (-1+5,-3)=(4,-3)`

Hence, the minimum point on the graph of the equation
`y=f(x+5)` =(4,-3)

Answer 2

The minimum point is (4,-3)

Explanation:

we know that

If the new equation is

y=f(x-5)

then

The Rule of the translation is

(x,y) -----> (x+5,y)

That means ----> The translation is 5 units at right

so

(−1,−3) ----> (-1+5,-3)

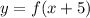(−1,−3) ----> (4,-3)