Answer:
m∠A = 39.5°
Explanation:
* Lets revise how to find the measure of an angle by using the cosine rule
- In any triangle ABC
# ∠A is opposite to side a
# ∠B is opposite to side b
# ∠C is opposite to side c
- The cosine rule is:
# a² = b² + c² - 2bc × cos(A)
# b² = a² + c² - 2ac × cos(B)
# c² = a² + b² - 2ab × cos(C)
- To find the angles use this rule
# m∠A =

# m∠B =
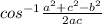
# m∠C =
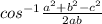
* Lets solve the problem
∵ a = 14 , b = 17 , c = 22
∵ m∠A =

∴ m∠A =
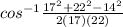
∴ m∠A =
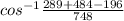
∴ m∠A =
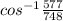
∴ m∠A = 39.5°