Question

In order to have $4,000 two years from now, how much would you have to put into an account today, if the interest rate is 4%, compounded quarterly? $______

Answer 1

We have to put $3693.44 into an account today.

Explanation:

From the given information it is clear that,

Amount = $4000.

Rate of interest = 4% compounded quarterly

Time = 2 years

We need to find the principle amount.

Let the principal amount be x.

Formula for amount in compound interest is

`A=P(1+(r)/(n))^(nt)` .... (1)

where,

P is principal money.

r is rate of interest.

n is number of time interest compounded in a period.

t is number of periods.

Substitute A=4000, r=0.04, n=4, t=2 in equation (1).

`4000=P(1+(0.04)/(4))^((4)(2))`

`4000=P((101)/(100)​)^(8)`

`4000=1.083P`

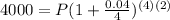
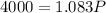
Divide both sides by 1.083 both sides.

`(4000)/(1.083)=P`

`P\approx 3693.44`

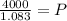
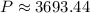
Therefore, we have to put $3693.44 into an account today.