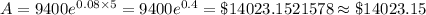Question

If $9,400 is invested at an interest rate of 8% per year, find the value of the investment at the end of 5 years if interested is compounded annually (once a year), semiannually (twice a year), monthly (12 times a year), daily (assume 365 days a year), or continuously. Round to the nearest cent. For each, use the correct compound interest formula from the following. A = P ( 1 + r n ) n t or A = P e r t

(a) Annual:

(b) Semiannual:

(c) Monthly:

(d) Daily:

(e) Continuously:

Answer 1

(a) $13811.68

(b) $13914.30

(c) $14004.55

(d) $14022.54

(e) $14023.15

Explanation:

Since, the amount formula in compound interest,

`A=P(1+(r)/(n))^(nt)`

Where,

P = Principal amount,

r = annual rate,

n = number of periods,

t = number of years,

Here, P = $ 9,400, r = 8% = 0.08, t = 5 years,

If the amount is compounded annually,

n = 1,

Hence, the amount of investment would be,

`A=9400(1+(0.08)/(1))^5=9400(1.08)^5=\$ 13811.6839219\approx \$ 13811.68`

(a) If the amount is compounded annually,

n = 1,

The amount of investment would be,

`A=9400(1+(0.08)/(1))^5=9400(1.08)^5=\$ 13811.6839219\approx \$ 13811.68`

(b) If the amount is compounded semiannually,

n = 2,

The amount of investment would be,

`A=9400(1+(0.08)/(2))^(10)=9400(1.04)^(10)=\$13914.2962782\approx \$ 13914.30`

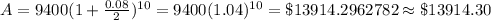
(c) If the amount is compounded Monthly,

n = 12,

The amount of investment would be,

`A=9400(1+(0.08)/(12))^(60)=9400(1+(1)/(150))^(60)=\$ 14004.549658\approx \$ 14004.55`

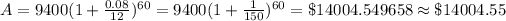
(d) If the amount is compounded Daily,

n = 365,

The amount of investment would be,

`A=9400(1+(0.08)/(365))^(365* 5)=9400(1+(2)/(9125))^(1825)=\$ 14022.5375476\approx \$ 14022.54`

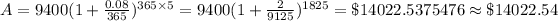
(e) Now, the amount in compound continuously,

`A=Pe^(rt)`

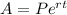
Where, P = principal amount,

r = annual rate,

t = number of years,

So, the investment would be,

`A=9400 e^(0.08* 5)=9400 e^(0.4)=\$14023.1521578\approx \$14023.15`