Answer:
He invest for 2 years.
Explanation:
Given : Suppose you have $1,950 in your savings account at the end of a certain period of time. You invested $1,700 at a 6.88% simple annual interest rate.
To find : How long, in years, did you invest your money?
Solution :
Applying simple interest formula,
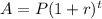
Where, A is the amount A=$1950
P is the principal P=$1700
r is the interest rate r=6.88%=0.0688
t is the time
Substitute the values in the formula,

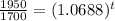
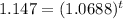
Taking log both side,
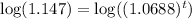
Applying logarithmic formula,


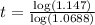

Approximately, He invest for 2 years.