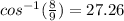Question

Find the intersection point and the angle between the lines L1 and L2. L1: x = - l + 2t, y = 3 - t, z = 2 + 2t, L2: x = - 2 - t, y = 5 + 2t, z = - 2t.

Answer 1

(-1,3,2)

Explanation:

For L1:

x= -1+2t

y= 3-t

z= 2+2t

and for L2:

x= -2-s

y= 5+2s

z=-2s

To find the intersection point we equalize the x, y and z:

-1+2t = -2-s

3-t = 5+2s

2+2t = -2s⇒ s= -1-t

We replace the s value -1-t in second equation:

3-t = 5+2(-1-t)

3-t = 5-2-2t

t = 0.

So, s = -1-t = -1-0 = -1.

We replace the s and t value in the parametric equations to find the interception point:

x= -1 +2(0) = -1

y = 3-0 = 3

z = 2 +2(0) = 2.

So, the interception point is (-1,3,2). The formula to calculate the angle is in the picture below, where α is the angle, u and v are the parallel vector of each line.

For L1: u=(2,-1,2) (the coefficients of t)

For L2: v=(-1,2,-2) (the coefficients of s)

So, the angle is:

cos(α) =
`\frac{\sqrt{2^(2)+(-1)^(2)+2^(2)}\sqrt{(-1)^(2)+2^(2)+(-2)^(2)}  }`

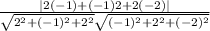
cos(α)=
`(|-2-2-4|)/(√(4+1+4)√(1+4+4))`

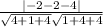
cos(α)=
`(|-8|)/(√(9)√(9))`

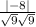
cos(α)=
`(8)/(9)`

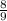
α =
`cos^(-1)((8)/(9))= 27.26`