Answer:
1.2 N, toward the center of the circle
Step-by-step explanation:
It is given that,
Mass of the ball, m = 0.015 kg
The radius of the circle, r = 0.7 m
Time taken by the ball to complete complete circle, t = 0.6 s
We need to find the tension in the string and its direction that provides the centripetal force acting on the ball to keep it in the circular path. Here, tension in the string balances the centripetal force so that the ball moves in circular path. So,

Since,
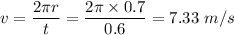
So,
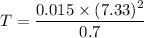
T = 1.15 N
or
T = 1.2 N
The direction of centripetal force is toward the center of circle. So, the correct option is (b).