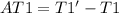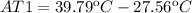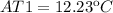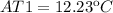Answer:
The temperature of the high-temperature source must increase in 12.23ºC.
Step-by-step explanation:
For a Carnot engine, the efficiency is defined as:
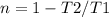
Where T2 and T1 are the low and the high-temperature sources respectively. Therefore for the value of T2 of 19.1ºC and the n equal to 30.7% (0.307), the T1 Temperature can be calculated as:
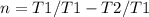
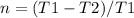
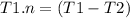
![T1-T1.n =T2[\tex]</p><p>[tex] T1(1-n) =T2](https://img.qammunity.org/2020/formulas/engineering/college/isxhh9qezkkei76q7i0e6yjie0knquif0z.png)
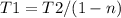
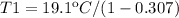
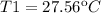
Then for the new effciencie n' of 52% (0.52) the new temeperature T1' will be:
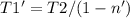

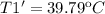
Finally the increment of temperature is: