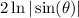Question

Can someone please help prove b.,c., and d.? i need help!!!

Answer 1

Proofs are in the explanation.

Explanation:

b) My first thought is to divide top and bottom on the left hand side by
`\cos(\alpha)`.

I see this would give me 1 on top and where that sine is, it would give me tangent since sine/cosine=tangent.

Let's do it and see:

`(\cos(\alpha))/(\cos(\alpha)-\sin(\alpha)) \cdot ((1)/(\cos(\alpha)))/((1)/(\cos(\alpha)))`

`=((\cos(\alpha))/(\cos(\alpha)))/((\cos(\alpha))/(\cos(\alpha))-(\sin(\alpha))/(\cos(\alpha)))`

`=(1)/(1-\tan(\alpha))`

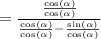
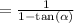
c) My first idea here is to expand the cos(x+y) using the sum identity for cosine.

So let's do that:

`(\cos(x)\cos(y)-\sin(x)\sin(y))/(\cos(x)\sin(y))`

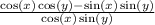
Separating the fraction:

`(\cos(x)\cos(y))/(\cos(x)\sin(y))-(\sin(x)\sin(y))/(\cos(x)\sin(y))`

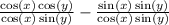
The cos(x) cancel's in the first fraction and the sin(y) cancels in the second fraction:

`(\cos(y))/(\sin(y))-(\sin(x))/(\cos(x))`

`\cot(y)-\tan(x)`

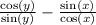
d) This one makes me think it is definitely essential that we use properties of logarithms.

The left hand side can be condense into one logarithm using the product law:

`\ln|(1+\cos(\theta))(1-\cos(\theta))|`

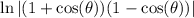
We are multiplying conjugates inside that natural log so we only need to multiply the first and the last:

`\ln|1-\cos^2(\theta)|`

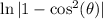
I can rewrite
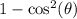`1-\cos^2(\theta)` using the Pythagorean Identity:

`\sin^2(\theta)+\cos^2(\theta)=1`:

`\ln|\sin^2(\theta)|`

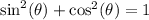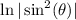
Now by power rule for logarithms:

`2\ln|\sin(\theta)|`