Question

At 85°C, the vapor pressure of A is 566 torr and that of B is 250 torr. Calculate the composition of a mixture of A and B that boils at 85°C when the pressure is 0.60 atm. Also, calculate the composition of the vapor mixture. Assume ideal behavior.

Answer 1

Composition of the mixture:

`x_(A) =0.652=65.2` %

`x_(B) =0.348=34.8` %

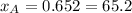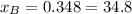Composition of the vapor mixture:

`y_(A) =0.809=80.9`%

`y_(B) =0.191=19.1`%

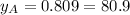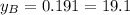Step-by-step explanation:

If the ideal solution model is assumed, and the vapor phase is modeled as an ideal gas, the vapor pressure of a binary mixture with
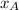`x_(A)` and
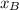`x_(B)` molar fractions can be calculated as:

`P_(vap)=x_(A)P_(A)+x_(B)P_(B)`

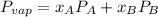
Where
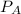`P_(A)` and
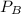`P_(B)` are the vapor pressures of the pure compounds. A substance boils when its vapor pressure is equal to the pressure under it is; so it boils when
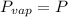`P_(vap)=P`. When the pressure is 0.60 atm, the vapor pressure has to be the same if the mixture is boiling, so:

`0.60*760=P_(vap)=x_(A)P_(A)+x_(B)P_(B)\\456=x_(A)P_(A)+(1-x_(A))P_(B)\\456=x_(A)*(P_(A)-P_(B))+P_(B)\\(456-P_(B))/(P_(A)-P_(B))=x_(A)\\\\(456-250)/(566-250)=x_(A)=0.652`

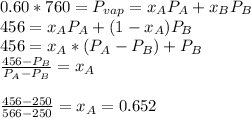
With the same assumptions, the vapor mixture may obey to the equation:

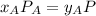`x_(A)P_(A)=y_(A)P`, where P is the total pressure and y is the fraction in the vapor phase, so:

`y_(A) =(x_(A)P_(A))/(P)=(0.652*566)/(456) =0.809=80.9` %

The fractions of B can be calculated according to the fact that the sum of the molar fractions is equal to 1.