Question

An 84.0 kg sprinter starts a race with an acceleration of 1.76 m/s2. If the sprinter accelerates at that rate for 11 m, and then maintains that velocity for the remainder of the 100 m dash, what will be his time (in s) for the race?

Answer 1

t=17.838s

Step-by-step explanation:

The displacement is divided in two sections, the first is a section with constant acceleration, and the second one with constant velocity. Let's consider the first:

The acceleration is, by definition:

`a=(dv)/(dt)=1.76`

So, the velocity can be obtained by integrating this expression:

`v=1.76t`

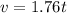
The velocity is, by definition:
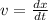`v=(dx)/(dt)`, so

`dx=1.76tdt\\x=1.76(t^(2))/(2)`.

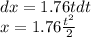Do x=11 in order to find the time spent.

`11=1.76(t^2)/(2)\\ t^2=(2*11)/(76) \\t=√(12.5)=3.5355s`

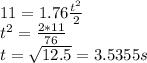
At this time the velocity is:
`v=1.76t=1.76*3.5355s=6.2225(m)/(s)`

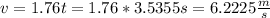
This velocity remains constant in the section 2, so for that section the movement equation is:

`x=v*t\\t=(x)/(v)`

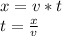
The left distance is 89 meters, and the velocity is
`6.2225(m)/(s)`, so:

`t=(89)/(6.2225)=14.303s`

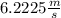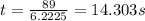
So, the total time is 14.303+3.5355s=17.838s