Question

Which term can be used in the blank of 36x3−22x2−__ so the greatest common factor of the resulting polynomial is 2x? Select two options

Answer 1

The 2nd and 3rd options. 4xy and 12x

Explanation:

I just took this test

Answer 2

The missing term is 4x

The missing term is 16x

Explanation:

* Lets explain how to solve the problem

- The polynomial is 36x³ - 22x² - ..............

- We need to find the missing term such that the greatest common

factor of the resulting polynomial is 2x

∵ The greatest power of x is 3, and there is another term with x²,

then the missing term must have x to have a greatest common

factor of the variable = x

∵ The coefficients of the given terms are 36 and 22 , the greatest

common factor of them is 2 so we must to chose the coefficient

of the missing term with a factor 2

# Option 1:

∵ the missing term is 4x

∵
`(36x^(3))/(2x)=18x^(2)`

∵
`(22x^(2))/(2x)=11x`

∵
`(4x)/(2x)=2`

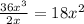
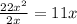
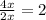
∴ 36x³ - 22x² - 4x = 2x(18x² - 11x - 2)

* The missing term is 4x

# Option 2:

∵ the missing term is 16x

∵
`(36x^(3))/(2x)=18x^(2)`

∵
`(22x^(2))/(2x)=11x`

∵
`(16x)/(2x)=8`

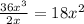
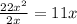
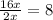
∴ 36x³ - 22x² - 16x = 2x(18x² - 11x - 8)

* The missing term is 16x