Answer:
1.5 meters.
Explanation:
The ladder is 4 meters.
It reaches the height of 3.7 meters on a wall.
This creates a right angled triangle of hypotenuse (4 meters) and height (3.7 meters).
We are to find the base length.
Applying the Pythagorean theorem;
a² + b² = c² , where a is the base length, b is the height length and c is the hypotenuse length.
∴ a² = c² - b² = 4² - 3.7² = 2.31
a (base length) =
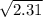 = 1.519868415 meters
= 1.519868415 meters
To round off the answer to the nearest tenth of a meter;
Tenth of a meter =
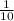 × 1 meter = 0.1 meters
× 1 meter = 0.1 meters
So we're rounding off the answer to one decimal place.
I.e 1.519868415 meters = 1.5 meters (rounded off to the nearest tenth of a meter.