Question

The graphs below have the same shape. What is the equation of the red graph?

Answer 1

c

Explanation:

since the axis of symmetry is 0, and the y intercept is 2, the answer should be c

Answer 2

Answer: The correct option is

(C)
`g(x)=x^2+2.`

Step-by-step explanation: Given that both the graphs shown have the same shape.

We are to find the equation of the red graph.

From the picture, we note that the equation of the blue graph is

`f(x)=x^2~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~(i)`

Also, the red graph is 2 units above the blue graph.

So, f(x) will be replaced by g(x) - 2 o find the equation for the red graph.

Therefore, we get from equation (i) that

`g(x)-2=x^2\\\\\Rightarrow g(x)=x^2+2.`

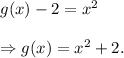
Thus, the required equation of the red graph is
`g(x)=x^2+2.`

Option (C) is CORRECT.