Question

Find the X intercept and the Y intercept of the graph of the equation. 9/8x+8y=18

Answer 1

(16, 0) and (0,
`(9)/(4)`)

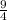Explanation:

Given

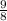`(9)/(8)` x + 8y = 18

Multiply through by 8 to eliminate the fraction

9x + 64y = 144

When the graph crosses the x- axis the y- coordinate of the point is zero.

Let y = 0 in the equation and solve for x

9x +0 = 144

9x = 144 ( divide both sides by 9 )

x = 16 ← x - intercept ⇒ (16, 0 )

When the graph crosses the y- axis the x- coordinate of the point is zero

let x = 0 in the equation and solve for y

0 + 64y = 144

64y = 144 ( divide both sides by 64 )

y =
`(144)/(64)` =
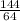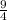`(9)/(4)` ← y- intercept ⇒ (0,
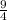`(9)/(4)` )

Answer 2

`\large\boxed{x-intercept=16\to(16,\ 0)}\\\boxed{y-intercept=(9)/(4)\to\left(0,\ (9)/(4)\right)}`

Explanation:

`(9)/(8)x+8y=18\\\\x-intercept\ \text{is for}\ y=0:\\\\(9)/(8)x+8(0)=18\\\\(9)/(8)x+0=18\\\\(9)/(8)x=18\qquad\text{multiply both sides by}\ (8)/(9)\\\\(8\!\!\!\!\diagup^1)/(9\!\!\!\!\diagup_1)\cdot(9\!\!\!\!\diagup^1)/(8\!\!\!\!\diagup_1)x=(8)/(9\!\!\!\!\diagup_1)\cdot18\!\!\!\!\!\diagup^2\\\\x=16\\\\y-intercept\ \text{is for}\ x=0:\\\\(9)/(8)(0)+8y=18\\\\0+8y=18\\\\8y=18\qquad\text{divide both sides by 8}\\\\y=(18)/(8)\\\\y=(9)/(4)`