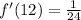Answer:
1/24
Explanation:
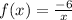
We want to find the derivative of f at x=12.
I'm assuming you want to see the formal definition of a derivative approach.
The definition is there:
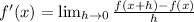
So we need to find f(x+h) given f(x).
To do this all you have to is replace old input, x, with new input, (x+h).
Let's do that:
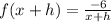 .
.
Let's go to the definition now:
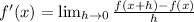
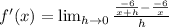
Multiply top and bottom by the least common multiple the denominators of the mini-fractions. That is, we are going to multiply top and bottom by x(x+h):
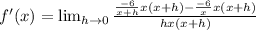
Let's cancel the (x+h)'s in the first mini-fraction.
We will also cancel the (x)'s in the second-mini-fraction.
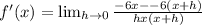
--=+ so I'm rewriting that part:
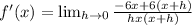
Distribute (NOT ON BOTTOM!):
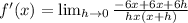
Simplify the top (-6x+6x=0):

Simplify the fraction (h/h=1):
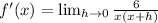
Now you can plug in 0 for h because it doesn't give you 0/0:

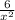
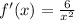
We want to evaluated the derivative at x=12 so replace x with 12:

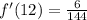
Divide top and bottom by 6: