Question

Find an equation of the line passing through the pair of points. Write the equation in the form Ax +By (5.3) and (4.1) C The equation of the line in the form Ai+By Cis (Simplily your answer Useintegers or fractions for any numbers in the equation) Emer your answer in the answer box

Answer 1

`2x-y=7`

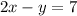
Explanation:

Here we are given two coordinates through which our lines passes through. Now we are going to use the two point form to find the equation of the line.

The two point form is given as

`(y-y_1)/(x-x_1)=(y_2-y_1)/(x_2-x_1)`

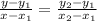
Here we are given two coordinates . Thus replacing them in the formula and simplifying it will give us the equation of the line.

`(y-1)/(x-4)=(3-1)/(5-4)`

`(y-1)/(x-4)=(2)/(1)`

`(y-1)=2(x-4)`

`y-1=2x-8`

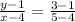
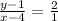
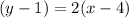
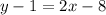
subtracting y and adding 8 on both sides we get

`2x-y=7`

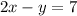
Hence this is our equation of the line passing through (5,3) & (4,1)