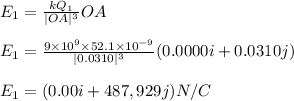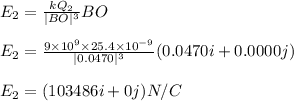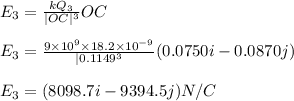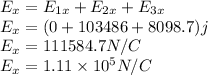Answer:
E= 1.11 x 10^5 N/C
Step-by-step explanation:
Given
Magnitude of charges
Q1 = -52.1 nC
Q2 = +25.4 nC
Q3 = -18.2 nC
Location of point Charge Q1, A= (0.00 i + 3.10 j) cm
Location of point charge Q2, B = (-4.71 i + 0.00 j) cm
Location of point charge Q3, C = (7.50 i -8.70 j) cm
location of the origin is O = (0.00 i + 0.00 j) cm
Solution
The eleectric feild will be directed away from the positive charge along the line BO and towards the negative charges along lines OA and OC respectively
OA = A -O = (0.00 i + 3.10 j) cm = (0.0000 i + 0.0310 j ) m ( direction of the
BO = O - B = (4.71 i - 0.00 j) cm = (0.0471 i + 0.000 j ) m
OC = C -A = (7.50 i -8.70 j) cm = (0.0750 i + 0.0870 j ) m