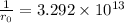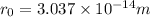Question

Rutherford discovered the nucleus of the atom by firing a particles at gold foil. An a particle has a charge of q = +2e and a mass of m = 6.64-10-27 kg. A gold nucleus has charge of Q-+79e. You may ignore the motion of the gold nucleus in this problem +2e +79e Suppose an α particles is traveling directly toward a gold nucleus. If the speed of the a particle is u = 1.9. 107 m/s when it is 1 m from the gold nucleus, how close to the gold nucleus will the a particle come before it stops and reverses direction?

Answer 1

Answer:
`r_0=3.037* 10^(-14)m`

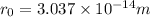
Step-by-step explanation:

Given

charge on alpha particle=+2e

mass of alpha particle=
`6.64* 10^(-27)` kg

Charge on gold nucleus=+79e

Velocity at r=1m is
`1.9* 10^(7)`

Using Energy conservation

Kinetic energy of particle will be converting to Potential energy as it approaches to nucleus

therefore

`(1)/(2)mv^2+U_(r=1m)=U_(closest\ to\ nucleus)`

`(1)/(2)\left ( 6.64* 10^(-27)\right )\left ( 1.9* 10^(7)^2\right )+(K\left ( 2e\right )\left ( 79e\right ))/(1)=(K\left ( 2e\right )\left ( 79e\right ))/(r_0)`

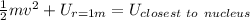
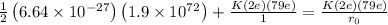
`(1)/(2)\left ( 6.64* 10^(-27)\right )\left ( 1.9* 10^(7)^2\right )=(9* 10^9* 158* \left ( 1.6* 10^(-19)\right ))/(y)\left [(1)/(r_0)-(1)/(1)\right ]`

on solving we get

`(1)/(r_0)=3.292* 10^(13)`

`r_0=3.037* 10^(-14)m`