Question

A 0.473 kg ice puck, moving east with a speed of 2.76 m/s, has a head-on collision with a 0.819 kg puck initially at rest. Assuming a perfectly elastic collision, what will be the speed and direction of each object after the collision?

Answer 1

The final speed of puck 1 is 0.739 m/s towards west and puck 2 is 2.02 m/s towards east .

Step-by-step explanation:

Let us consider east as positive direction and west as negative direction .

Given

mass of puck 1 ,
`m_1= 0.473 kg`

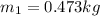
mass of puck 2 ,
`m_2= 0.819 kg`

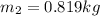
initial speed of puck 1 ,
`u_1=2.76(m)/(s)`

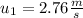
initial speed of puck 2 ,
`u_2=0.00(m)/(s)`

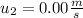
Final speed of puck 1 and puck 2 be
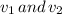`v_1\, and\, v_2` respectively

Apply conservation of linear momentum

`m_1u_1+m_2u_2=m_1v_1+m_2v_2`

=>
`0.473* 2.76+0.0=0.473* v_1+0.819* v_2`

=>
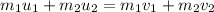
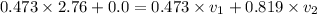
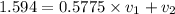`1.594=0.5775* v_1+ v_2` -----(A)

Since collision is perfectly elastic , coefficient restitution e=1

`u_2-u_1=v_1-v_2`

=>
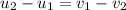
`0-2.76=v_1-v_2` ------(B)

From equation (A) and (B)

`v_1=-0.739(m)/(s)`

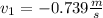
and
`v_2=2.02(m)/(s)`

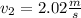
Thus the final speed of puck 1 is 0.739 m/s towards west and puck 2 is 2.02 m/s towards east .