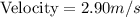Question

When a bucket of water is swung in a vertical circle, the water will remain in the bucket if the velocity is kept high enough. If you let the bucket slow down too much, the water will spill out. The critical velocity is the slowest velocity necessary to keep the water in the bucket.What is the critical velocity if the formula is: Velocity = SQRT (R)(G)Where the radius of your arm swing including the bucket is r and g is the acceleration of gravity?Hint: You must measure or estimate the length from your shoulder to the center of the bucket to determine the radius.Variables:My arm is 28 inches longmy bucket is 12 inches.

Answer 1

`\textup{Velocity} = 2.90 m/s`

Step-by-step explanation:

Given:

The formula for the critical velocity as:

`\textup{Velocity} = √(RG)`

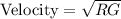
where,

G is mentioned as acceleration due to gravity = 9.8 m/s²

Radius of the arm, R₁ = 28 inches = 28 × 0.0254 = 0.7112 m [as 1 in = 0.0254 m]

Length of the bucket, L₂ = 12 in = 12 × 0.0254 = 0.3048 m

now, the radius of the bucket upto its center, R₂ = L₂/2 = 0.3048/2 = 0.1524 m

Total radius = R₁ + R₂ = 0.7112 m + 0.1524 m = 0.8636 m

on substituting the value in the formula for the velocity, we get

`\textup{Velocity} = √(0.8636* 9.8)`

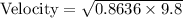
or

`\textup{Velocity} = 2.90 m/s`