Step-by-step explanation:
Let f₁ is the fundamental frequency,
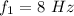
Lower pitch frequency,

Fundamental frequency is,
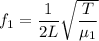 .....(1)
.....(1)
Lower frequency is,
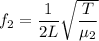 ..............(2)
..............(2)
Dividing equation (1) and (2) as :
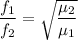
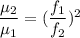
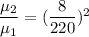

So, the ratio of linear mass density μ of the string with the higher pitch to that of the string with the lower pitch is 0.00132. Hence, this is the required solution.