Question

A different particle moves in 3 dimensional space. Its velocity is v = {t^2i + 2t^2j + 3 rk} in [m/s]. Find the magnitude of its acceleration at t = 3 s

Answer 1

Step-by-step explanation:

It is given that,

Velocity of the particle is given by:

`v=(t^2i+2t^2j+3tk)`

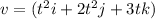
We need to find the magnitude of its acceleration at t = 3 seconds. We know that, acceleration a is given by :

`a=(dv)/(dt)`

`a=(d(t^2i+2t^2j+3tk))/(dt)`

`a=(2t\ i+4t\ j+3\ k)`

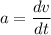
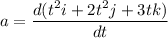
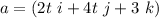
At t = 3 seconds

`a=(2(3)\ i+4(3)\ j+3\ k)`

`a=6\ i+12\ j+3\ k`

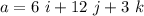
So, the magnitude of a is given by :

`|a|=√(6^2+12^2+3^2)`

`a=13.7\ m/s^2`

So, the magnitude of acceleration is 13.7 m/s². Hence, this is the required solution.