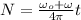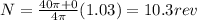Answer:
Part a)
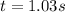
Part b)
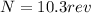
Step-by-step explanation:
Mass of the disc is given as
m = 2.00 kg
radius of the disc is given as
r = 7 cm
so moment of inertia of the disc is given as
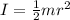
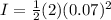

Now given that torque on the disc is
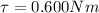
so here the angular acceleration is given as
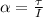

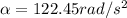
Part a)
if disc start from rest and then achieve final speed as 1200 rpm then
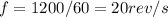
so final speed is

now the time taken to reach this speed is given as
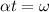
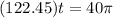
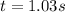
Part b)
Number of revolution in the same time is given as