Question

An object is launched upward from 62.5 meters above ground level with an initial velocity of 12 meters per second. The gravitational pull of the earth is about 4.9 meters per second squared. How long will the object take to hit the ground? 5) Explain which model would you would choose and why.An object is launched upward from 62.5 meters above ground level with an initial velocity of 12 meters per second. The gravitational pull of the earth is about 4.9 meters per second squared. How long will the object take to hit the ground? 5) Explain which model would you would choose and why.

Answer 1

5 seconds

Explanation:

This follows the pattern

`h(t)=-4.9t^2+v_(0)t+h_(0)`

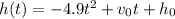
It is parabolic and it is used to model projectile motion. This is the model you would use. Now for the math of it.

The v₀ is the initial velocity and the h₀ is the initial height. The whole thing is negative because it is an upside down parabola. Our initial velocity is 12 and the initial height is 62.5. That means that our particular model is

`h(t)=-4.9t^2+12t+62.5`

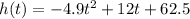
h(t) is the height of the projectile after a certain length of time, t, has gone by. We want to know how long, t, it takes the projectile to hit the ground. When something is laying on the ground, its height is 0. Therefore, in order to find how long it takes for the height to be 0, we replace h(t) with 0 and then factor to find the values of t:

`0=-4.9t^2+12t+62.5`

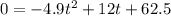
If you plug this into the quadratic formula you will get that the values of t are

t = -2.55 and t = 5

We all know that the 2 things in math that will never EVER be negative are time and distance/measures, so we can disregard the negative value of time and say that the length of time it takes for the object to hit the ground from its initial height of 62.5 m is 5 seconds.