Answer:
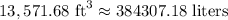
Explanation:
We are asked to find the amount of water that the well can hold.
We will use volume of cylinder formula to solve our given problem.
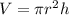 , where,
, where,
V = Volume of cylinder,
r = Radius,
h = Height.
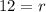
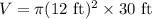
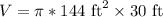
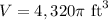
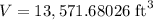

Since our given volume is in cubic feet, so we can convert it in liters by multiplying 13,571.68 by 28.3168.
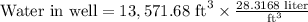
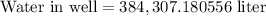
Therefore, the well could hold approximately 384307.18 liters of water.