Answer:
11)
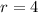
12)
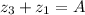
13a)
 and
and
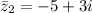
13b)

Explanation:
11. The given equation in rectangular coordinates is
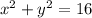
To convert to polar form, use the relation
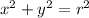
This implies that:

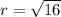
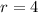
In polar coordinates,
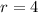 is a circle with radius 4 units.
is a circle with radius 4 units.
12. From the diagram;

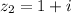
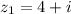
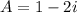
We can see that two arrows are moving in anticlockwise direction to meet A which is moving in the clockwise direction;

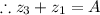
13. The conjugate of the complex number:
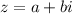 is
is
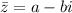
Part a) The given complex numbers are:
 and
and
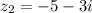
The conjugates of these complex numbers are:
 and
and
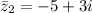
Part b) The product of
 and
and
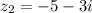 is
is
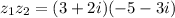


The conjugate of the product is:
