Answer:
The rate of ladder moving down against the wall is 1.125 ft/s
Step-by-step explanation:
Note: Refer to the attached figure
Applying the concept of the Pythagoras we have
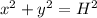
or
9²+y² = 15²
⇒y² = 225 - 81
⇒y = √144 = 12 m
also,
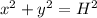
differentiating with respect to time, we get
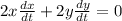
substituting the values in the above equation we get
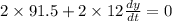
or

or
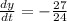
or
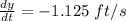
here, the negative sign depicts that the value of y is decreasing or the ladder is moving down.
hence, the rate of ladder moving down against the wall is 1.125 ft/s