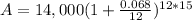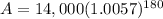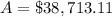Question

Say you have $14,000 to invest into an investment account. You can either invest your money into an account with a 7% annual interest rate which is compounded quarterly, or an account with a 6.8% annual interest rate which is compounded monthly, which should you choose for a 15-year investment?

Answer 1

You should choose an account with a 7% annual interest rate which is compounded quarterly

Explanation:

we know that

The compound interest formula is equal to

`A=P(1+(r)/(n))^(nt)`

where

A is the Final Investment Value

P is the Principal amount of money to be invested

r is the rate of interest in decimal

t is Number of Time Periods

n is the number of times interest is compounded per year

part 1)

we have

`t=15\ years\\ P=\$14,000\\ r=0.07\\n=4`

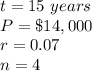
substitute in the formula above

`A=14,000(1+(0.07)/(4))^(4*15)`

`A=14,000(1.0175)^(60)`

`A=\$39,645.43`

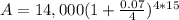
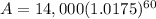
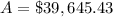
part 2)

we have

`t=15\ years\\ P=\$14,000\\ r=0.068\\n=12`

substitute in the formula above

`A=14,000(1+(0.068)/(12))^(12*15)`

`A=14,000(1.0057)^(180)`

`A=\$38,713.11`