Answer:
The speed of sound in this gas is 409.6 m/s.
Step-by-step explanation:
The length of the column changes from 20 cm from resonance to resonance. Thus,
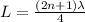
The length change from one resonance to resonance. so, there is 1 loop change. So,
ΔL = 1 loop = λ/2
ΔL = 20 cm (given)
Also, 1 cm = 0.01 m
So,
ΔL = 0.2 cm (given)
The wavelength is:
λ = ΔL×2
λ = 2x0.2 = 0.4 m
Given:
Frequency (ν) = 1024 Hz
Velocity of the sound in the gas = ν×λ = 1024×0.4 m/s = 409.6 m/s