Question

A neutron star and a white dwarf have been found orbiting each other with a period of 28 minutes. If their masses are typical, what is their average separation? Compare the separation with the radius of the sun, or about 0.005 AU. (Hints: Refer to Kepler's third law with regard to mass. Assume the mass of the neutron star is 2.5 solar masses and the mass of the white dwarf is 0.3 solar mass.)

Answer 1

The average separation is 0.002041 AU

Step-by-step explanation:

Given data:

Mass of the neutron star, M₁ = 2.5
`M_(solar)`

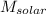
Mass of the White dwarf, M₂ = 0.3
`M_(solar)`

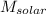
Orbiting period (P)= 28 minutes

1 year = 365 × 24 × 60 minutes = 525600 minutes

or

1 minute = 1/525600 years

thus, 28 minutes = 28/525600 years = 5.327 × 10⁻⁵ years

now from the Kepler's third law we have,

MP² = a³

where, P is the period

M is the mass = M₁ + M₂

a is the size of the orbit

thus, by substituting the values in the equation we get

(2.5
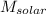`M_(solar)`+0.3
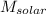`M_(solar)`)(5.327 × 10⁻⁵ years)² = a³

Also,

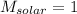`M_(solar)=1` when planets orbiting sun

thus,

2.8 ×(5.327 × 10⁻⁵ years)² = a³

or

a³ = 7.94 × 10⁻⁹

or

a = 1.99 × 10⁻³ AU

thus, the average separation is 0.001995 AU

Now

1 AU = 1.5 × 10⁸ km

thus,

0.001995 AU = 299281.61 km = 2.99 × 10⁵ km

in terms of sun's radius = (2.99 × 10⁵ km)/(7 ×10⁵) = 0.427

Thus, the this orbit system will fit inside the sun