For this case we must find the value of "x" of the following expression:
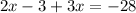
We add similar terms:

We add 3 to both sides of the equation:
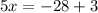
Different signs are subtracted and the sign of the major is placed:

We divide between 5 on both sides of the equation:
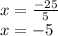
ANswer:
