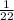Question

A random variable X follows the continuous uniform distribution with a lower bound of −4 and an upper bound of 18. a. What is the height of the density function f(x)

Answer 1

Height of density function is equal to
`(1)/(22)`.

Explanation:

Given that

Lower bound= -4 and upper bound=18 and we need to find height of density function.

We know that height ofProbability density function given as

`Height =(1)/(Upper\ bound -lower\ bound)`

Now by putting the values in the above formula we will get

`Height =(1)/(18 -(-4))`

`Height =(1)/(22)`

So height of density function is equal to
`(1)/(22)`.

Answer 2

The height of the density function is
`(1)/(22)`

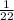
Explanation:

Given : A random variable X follows the continuous uniform distribution with a lower bound of −4 and an upper bound of 18.

To find : What is the height of the density function f(x)?

Solution :

According to question,

The height of the density function is given by,

`f(X)=(1)/(b-a)`

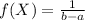
Where, a is the lower bound a=-4

b is the upper bound b=18

Substitute the value in the formula,

`f(X)=(1)/(18-(-4))`

`f(X)=(1)/(18+4)`

`f(X)=(1)/(22)`

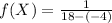
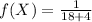
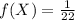
Therefore, The height of the density function is
`(1)/(22)`