Question

When 0.6943 g of a compound is subjected to combustion analysis it produced 1.471 g CO2 and 0.391 g H2O. What is its empirical and molecular formula if its molar mass is 172 g/mol if the compound is composed of only carbon, hydrogen, and oxygen.

Answer 1

To find the empirical formula, calculate the moles of carbon and hydrogen using the masses of CO2 and H2O produced during combustion. The empirical formula of the compound is CH. The molecular formula is C12H12.

Step-by-step explanation:

To find the empirical formula of the compound, first, calculate the moles of carbon and hydrogen using the masses of CO2 and H2O produced during combustion. The molar amounts can be determined by dividing the masses by their respective molar masses. In this case, 1.471 g CO2 is equivalent to 0.034 mol C, and 0.391 g H2O is equivalent to 0.022 mol H.

The ratio between C and H in the compound can then be determined by dividing the moles of C and H by the smaller value, which is 0.022 mol H. This gives a ratio of approximately 1.545 mol C to 1 mol H. Since the ratio is close to 1:1, the empirical formula of the compound is CH.

To determine the molecular formula, divide the molar mass of the compound (172 g/mol) by the empirical mass of CH (14.03 g/mol). The result is approximately 12.27, which rounds to 12. This means the molecular formula is (CH)12, or C12H12.

Answer 2

Answer: The empirical molecular formula for the given organic compound are
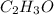`C_2H_3O` and
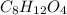`C_8H_(12)O_4` respectively.

Step-by-step explanation:

The chemical equation for the combustion of hydrocarbon having carbon, hydrogen and oxygen follows:

`C_xH_yO_z+O_2\rightarrow CO_2+H_2O`

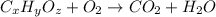
where, 'x', 'y' and 'z' are the subscripts of Carbon, hydrogen and oxygen respectively.

We are given:

Mass of
`CO_2=1.471g`

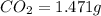
Mass of
`H_2O=0.391g`

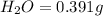
We know that:

Molar mass of carbon dioxide = 44 g/mol

Molar mass of water = 18 g/mol

• For calculating the mass of carbon:

In 44g of carbon dioxide, 12 g of carbon is contained.

So, in 1.471 g of carbon dioxide,
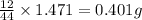`(12)/(44)* 1.471=0.401g` of carbon will be contained.

• For calculating the mass of hydrogen:

In 18g of water, 2 g of hydrogen is contained.

So, in 0.391 g of water,
`(2)/(18)* 0.391=0.0434g` of hydrogen will be contained.

• Mass of oxygen in the compound = (0.6943) - (0.401 + 0.0434) = 0.2499 g

To formulate the empirical formula, we need to follow some steps:

• Step 1: Converting the given masses into moles.

Moles of Carbon =
`\frac{\text{Given mass of Carbon}}{\text{Molar mass of Carbon}}=(0.401g)/(12g/mole)=0.0334moles`

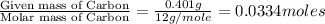
Moles of Hydrogen =
`\frac{\text{Given mass of Hydrogen}}{\text{Molar mass of Hydrogen}}=(0.0434g)/(1g/mole)=0.0434moles`

Moles of Oxygen =
`\frac{\text{Given mass of oxygen}}{\text{Molar mass of oxygen}}=(0.2499g)/(16g/mole)=0.0156moles`

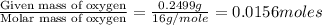
• Step 2: Calculating the mole ratio of the given elements.

For the mole ratio, we divide each value of the moles by the smallest number of moles calculated which is 0.0156 moles.

For Carbon =
`(0.0334)/(0.0156)=2.14\approx 2`

For Hydrogen =
`(0.0434)/(0.0156)=2.78\approx 3`

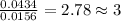
For Oxygen =
`(0.0156)/(0.0156)=1`

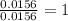
• Step 3: Taking the mole ratio as their subscripts.

The ratio of C : H : O = 2 : 3 : 1

Hence, the empirical formula for the given compound is
`C_2H_(3)O_1=C_2H_3O`

• For determining the molecular formula, we need to determine the valency which is multiplied by each element to get the molecular formula.

The equation used to calculate the valency is :

`n=\frac{\text{molecular mass}}{\text{empirical mass}}`

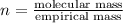
We are given:

Mass of molecular formula = 172 g/mol

Mass of empirical formula = 43 g/mol

Putting values in above equation, we get:

`n=(172g/mol)/(43g/mol)=4`

Multiplying this valency by the subscript of every element of empirical formula, we get:

`C_((2* 4))H_((3* 4))O_((1* 4))=C_8H_(12)O_4`

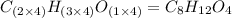
Thus, the empirical and molecular formula for the given organic compound are
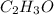`C_2H_3O` and
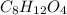`C_8H_(12)O_4` respectively.