Answer:
The distance between the two slits is 40.11 μm.
Step-by-step explanation:
Given that,
Frequency

Distance of the screen l = 88.0 cm
Position of the third order y =3.10 cm
We need to calculate the wavelength
Using formula of wavelength
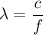
where, c = speed of light
f = frequency
Put the value into the formula
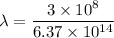
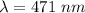
We need to calculate the distance between the two slits
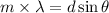
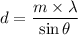
Where, m = number of fringe
d = distance between the two slits
Here,
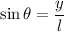
Put the value into the formula
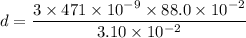
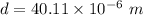
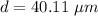
Hence, The distance between the two slits is 40.11 μm.