Question

7% of items in a shipment are known to be defective. If a sample of 5 items is randomly selected from this shipment, what is the probability that at least one defective item will be observed in this sample? Round your result to 2 significant places after the decimal (For example, 0.86732 should be entered as 0.87).

Answer 1

Answer: 0.30

Explanation:

Binomial distribution formula :-

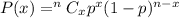`P(x)=^nC_xp^x(1-p)^(n-x)`, where P(x) is the probability of getting success in x trials , n is the total number of trials and p is the probability of getting success in each trial.

Given : The probability that a shipment are known to be defective= 0.07

If a sample of 5 items is randomly selected from this shipment,then the probability that at least one defective item will be observed in this sample will be :-

`P(X\geq1)=1-P(0)\\\\=1-(^5C_0(0.07)^0(1-0.07)^(5-0))\\\\=1-(0.93)^5=0.3043116307\approx0.30`

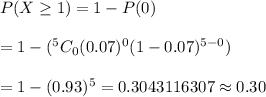
Hence, the probability that at least one defective item will be observed in this sample =0.30