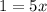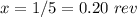Question

Charmaine is riding her bike. The distance she travels varies directly with the number of revolutions (turns) her wheels make. See the graph below.

(a) How many revolutions does Charmaine make per foot of distance traveled?
(b) What is the slope of the graph?

Answer 1

Part a) 0.20 revolutions per foot of distance traveled

Part b) The slope of the graph is
`m=5(ft)/(rev)`

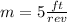
Explanation:

step 1

Find the slope

we know that

A relationship between two variables, x, and y, represent a proportional variation if it can be expressed in the form
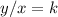`y/x=k` or
`y=kx`

In a proportional relationship the constant of proportionality k is equal to the slope m of the line and the line passes through the origin

Let

x ----> the number of revolutions

y ----> the distance traveled in feet

we have the point (2,10) ----> see the graph

Find the value of the constant of proportionality k

`k=y/x=10/2=5(ft)/(rev)`

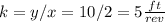
Remember that in a direct variation the constant k is equal to the slope m

therefore

The slope m is equal to

`m=5(ft)/(rev)`

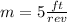
The linear equation is

`y=5x`

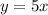
step 2

How many revolutions does Charmaine make per foot of distance traveled?

For y=1

substitute in the equation and solve for x

`1=5x`

`x=1/5=0.20\ rev`