Question

An automobile tire has a volume of 1.63 x 10-2 m3 and contains air at a gauge pressure (pressure above atmospheric pressure) of 165 kPa when the temperature is 0.00°C. What is the gauge pressure of the air in the tires when its temperature rises to 27.3°C and its volume increases to 1.70 x 10-2 m3

Answer 1

Answer: The gauge pressure of the air in the tires is 179.5 kPa.

Solution :

Combined gas law is the combination of Boyle's law, Charles's law and Gay-Lussac's law.

The combined gas equation is,

`(P_1V_1)/(T_1)=(P_2V_2)/(T_2)`

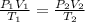
where,

`P_1` = initial pressure of gas = Atmospheric pressure + gauge pressure = 101 kPa + 165 kPa = 266 kPa

`P_2` = final pressure of gas = ?

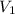`V_1` = initial volume of gas =
`1.63* 10^(-2)m^3`

`V_2` = final volume of gas =
`1.70* 10^(-2)m^3`

`T_1` = initial temperature of gas =
`0^oC=273+0=273K`

`T_2` = final temperature of gas =
`27.3^oC=273+27.3=300.3K`

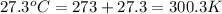
Now put all the given values in the above equation, we get the final pressure of gas.

`(266* 1.63* 10^(-2))/(273K)=(P_2* 1.70* 10^(-2))/(300.3K)`

`P_2=280.5kPa`

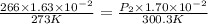
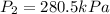
Gauge pressure = Absolute pressure - atmospheric pressure = (280.5 - 101) kPa= 179.5 kPa

Therefore, the gauge pressure of the air in the tires is 179.5 kPa.