Question

Find the linear function that models the town’s population P as a function of the year, t, where t is the number of years since the model began.

Answer 1

The equation is:

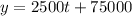`y=2500t+75000` where t is the number of years satisfying the inequality
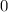`0\let\le5` and y is the population at that time.

Explanation:

So it gives us the initial amount 75000; this is what happens at time 0.

So we have the point (0,75000) is on the function's graph.

(0,75000)

(1,77500) -> It goes 2500 per year. So year 1 the population is 75000+2500.

So we have the y-intercept of the line which is (0,75000).

We just need the slope.

Our goal is to put into slope-intercept form: y=mt+b form where m is the slope and b is the y-intercept.

To find the slope, you line up the points vertically and subtract then put 2nd difference over 1st difference.

( 1 , 77500 )

-( 0 , 75000)

------------------------

1 2500

So the slope is 2500/1=2500 which we should have known earlier since the y's are increasing by 2500 while the x's are increasing by 1.

So while t is between 0 and 5 inclusive we have the following equation:

y=2500t+75000