Answer: The value of b = 0.99
The probability that a day requires more water than is stored in city reservoirs is 0.161.
Explanation:
Given : Water use in the summer is normally distributed with
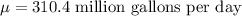
Standard deviation :
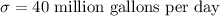
Let x be the combined storage capacity requires by the reservoir on a random day.
Z-score :
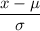
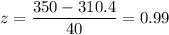
The probability that a day requires more water than is stored in city reservoirs is :
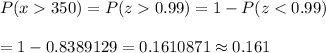
Hence, the probability that a day requires more water than is stored in city reservoirs is 0.161