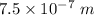Answer:
The wavelength of the monochromatic light is
Step-by-step explanation:
Given that,
Distance between the slits d = 1.8 mm
Distance of fringe from the slits D =4.8 m
Number of fringe m =1
Distance between the fringes = 1 cm
We need to calculate the wavelength of monochromatic light
Using formula of young's double slits
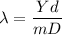
Where, d = Distance between the slits
D = Distance of fringe from the slits
m = Number of fringe
y = Distance between the fringes
Put the value in to the formula
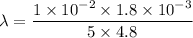
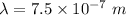
Hence, The wavelength of the monochromatic light is