Question

The tallest living man at one time had a height of 262 cm. The shortest living man at that time had a height of 68.6 cm. Heights of men at that time had a mean of 175.32 cm and a standard deviation of 8.17 cm. Which of these two men had the height that was more​ extreme?

Answer 1

The shortest living man's height was more extreme.

Explanation:

We have been given that the the tallest living man at one time had a height of 262 cm. The shortest living man at that time had a height of 68.6 cm. Heights of men at that time had a mean of 175.32 cm and a standard deviation of 8.17 cm.

First of all, we will find z-scores for both heights suing z-score formula.

`z=(x-\mu)/(\sigma)`

`z=(68.6-175.32)/(8.17)`

`z=(-106.72)/(8.17)`

`z=-13.06`

`z=(x-\mu)/(\sigma)`

`z=(262-175.32)/(8.17)`

`z=(86.68)/(8.17)`

`z=10.61`

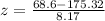
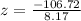
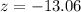
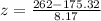
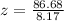
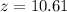
Since the data point with a z-score
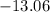`-13.06` is more away from the mean than data point with a z-score
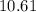`10.61`, therefore, the shortest living man's height was more extreme.

Answer 2

Answer:

more the z-score more will be the extreme. therefore tallest man has high extreme

Explanation:

Formula for z-score:
`(X-\mu )/(\sigma)`

where

X is height of tallest man

μ mean height

σ is standard deviation

z score for tallest is

z-score =
`( 262 - 175.32)/(8.17) = 10.60`

similarly for shortest man

z-score =
`(68.6 - 175.32)/(8.17) = - 13.06`

more the z-score more will be the extreme. therefore tallest man has high extreme