Question

What is the volume of the prism below 4 height length 18 9width

Answer 1

The volume of the prism is
`\(V = 648 \, \text{cubic units}\).`

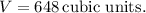
Step-by-step explanation:

The formula for the volume (V) of a rectangular prism is given by
`\(V = lwh\),` where (l) is the length, (w) is the width, and (h) is the height. In this case, the provided dimensions are length (l = 18), width (w = 9), and height (h = 4). Substituting these values into the formula:
`\[ V = 18 * 9 * 4 = 648 \, \text{cubic units}. \]` Therefore, the volume of the prism is
`\(648 \, \text{cubic units}\).`

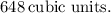
Understanding the concept of volume in three-dimensional geometry is vital. The formula
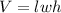`\(V = lwh\)` reflects the relationship between the length, width, and height of a rectangular prism. Multiplying these dimensions provides the total space enclosed by the prism in cubic units. In this instance, with a length of 18 units, a width of 9 units, and a height of 4 units, the volume calculation yields
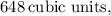`\(648 \, \text{cubic units}\),` representing the spatial capacity of the given prism.

Calculating the volume of prisms is a fundamental skill in geometry and has practical applications in various fields, including architecture and engineering. This calculation allows us to quantify the amount of space a three-dimensional object occupies, providing essential information for design and analysis. In this case, the resulting volume,
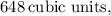`\(648 \, \text{cubic units}\),` represents the capacity of the rectangular prism specified by the given dimensions.

Answer 2

For this case we have that by definition, the volume of a prism is given by:

`V = a * b * c`

Where:

a: Long

b: Width

c: It is the height

According to the data we have:

`a = 18\\b = 9\\c = 4`

Substituting we have:

`V = 18 * 9 * 4\\V = 648`

Finally, the volume of the prism i
`648 \ units ^ 3`s 648 \ units ^ 3

Answer:

`648 \ units ^ 3`