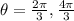Question

Solve sin 0 + 1 = cos20 on the interval 0 ≤ 0 < 2pi. Show work please!

Answer 1

`\theta=(\pi)/(2),(3\pi)/(2)(2\pi)/(3)(4\pi)/(3)`

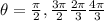
Explanation:

You need 2 things in order to solve this equation: a trig identity sheet and a unit circle.

You will find when you look on your trig identity sheet that

`cos(2\theta)=1-2sin^2(\theta)`

so we will make that replacement, getting everything in terms of sin:

`sin(\theta)+1=1-2sin^2(\theta)`

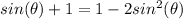
Now we will get everything on one side of the equals sign, set it equal to 0, and solve it:

`2sin^2(\theta)+sin(\theta)=0`

We can factor out the sin(theta), since it's common in both terms:

`sin(\theta)(2sin(\theta)+1)=0`

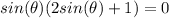
Because of the Zero Product Property, either

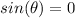`sin(\theta)=0` or

`2sin(\theta)+1=0`

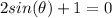
Look at the unit circle and find which values of theta have a sin ratio of 0 in the interval from 0 to 2pi. They are:

`\theta=(\pi)/(2),(3\pi)/(2)`

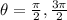
The next equation needs to first be solved for sin(theta):

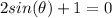`2sin(\theta)+1=0` so

`2sin(\theta)=-1` and

`sin(\theta)=-(1)/(2)`

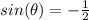
Go back to your unit circle and find the values of theta where the sin is -1/2 in the interval. They are:

`\theta=(2\pi)/(3),(4\pi)/(3)`