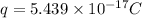Answer:
Step-by-step explanation:
Given:
Mass of the tiny sphere, M = 2.0 × 10⁻⁶ kg
also masses are equal i.e M₁ = M₂
Now,
the gravitational force between the two masses M₁ and M₂ is given as:
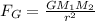
where,
G is the gravitational force constant = 6.67 x 10⁻¹¹ m³/kg.s²
r = center to center distance between the masses
also,
Electric force between the charges is given as
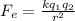
where,
q₁ and q₂ are the charges and also it is given that q₁=q₂=q
k is the coulomb's law constant = 9.0 x 10⁹ N.m²/C²
since it is mentioned that
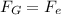
we have
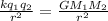
or
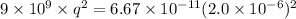
or