Answer: 0.9871
Explanation:
Given : A manufacturer knows that their items have a lengths that are approximately normally distributed with
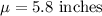
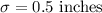
Sample size :
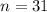
Let x be the length of randomly selected item.
z-score :
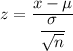
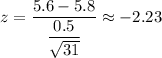
The probability that their mean length is greater than 5.6 inches by using the standard normal distribution table
=

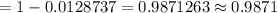
Hence, the probability that their mean length is greater than 5.6 inches is 0.9871.