Question

According to a recent​ study, 9.3​% of high school dropouts are​ 16- to​ 17-year-olds. In​ addition, 6.4​% of high school dropouts are white​ 16- to​ 17-year-olds. What is the probability that a randomly selected dropout is​ white, given that he or she is 16 to 17 years​ old?

Answer 1

0.688 or 68.8%

Explanation:

Percentage of high school dropouts = P(D) = 9.3% = 0.093

Percentage of high school dropouts who are white =
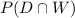`P(D \cap W)` = 6.4% = 0.064

We need to find the probability that a randomly selected dropout is​ white, given that he or she is 16 to 17 years​ old. This is conditional probability which can be expressed as: P(W | D)

Using the formula of conditional probability, we ca write:

`P(W | D)=(P(W \cap D))/(P(D))`

Using the values, we get:

P( W | D) =
`(0.064)/(0.093) = 0.688`

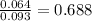
Therefore, the probability that a randomly selected dropout is​ white, given that he or she is 16 to 17 years​ old is 0.688 or 68.8%