Answer:
The slope of XZ is 3/4 , the slope of XY is -4/3 , and XZ = XY = 5 ⇒ 3rd answer
Explanation:
* Lets look to the attached figure to solve the problem
- To prove that the Δ XYZ is an isosceles right triangle, you must
find two sides the product of their slopes is -1 and they are equal
in lengths
- From the figure the vertices of the triangle are;
X = (1 , 3) , Y = (4 , -1) , Z = (5 , 6)
- The slope of the line whose endpoints are (x1 , y1) and (x2 , y2)
is
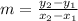
∵ The slope of
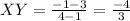
∵ The slope of
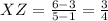
∴ The slope of XY = -4/3 , the slope of XZ = 3/4
∵ -4/3 × 3/4 = -1
∴ XY ⊥ XZ
∴ ∠ X is a right angle
∴ Δ XYZ is a right triangle
- The distance between the two points (x1 , y1) and (x2 , y2) is
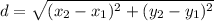
∵
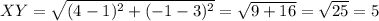
∵
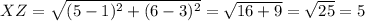
∴ XY = XZ = 5
∴ Δ XYZ is an isosceles right triangle
* The statement which prove that is:
The slope of XZ is 3/4 , the slope of XY is -4/3 , and XZ = XY = 5