Question

The mean pulse rate​ (in beats per​ minute) of adult males is equal to 69 bpm. For a random sample of 159 adult​ males, the mean pulse rate is 70.3 bpm and the standard deviation is 10.6 bpm. Find the value of the test statistic.

Answer 1

1.55

Explanation:

Mean pulse rate of adults = u = 69 bpm

Sample size = n = 159

Mean pulse rate of sample = x = 70.3 bpm

Standard Deviation of sample = s = 10.6 bpm

We have to find the test statistic. Since the sample size is larger than 30 we can assume that the population is normally distributed. We have the sample standard deviation, so we will use the t-distribution to solve this problem. z-distribution would have been used if the value of population standard deviation was known.

The t-test statistic is calculated as:

`t =(x-u)/((s)/(√(n)))`

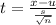
Using the values, we get:

`t=(70.3-69)/((10.6)/(√(159)))\\\\ t = 1.55`

Thus, the value of t-test statistic would be 1.55